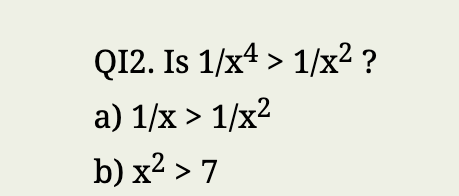As most GMAT Test takers already know, this is a very specific type GMAT Inequalities problems that they often come across while practicing GMAT Math. Quite frequently, test takers either get this type of GMAT Inequalities problems incorrect (thus loosing points) or spend too much time on them resulting in time wastage.
QI2. Is 1/x4 > 1/x2 ?
a) 1/x > 1/x2
b) x2 > 7
Hence, to make it quick and easy to solve these type of problems, we have developed a GMAT Inequalities Comparison Framework. However, the framework does come with a caveat – you must remember the range table or must atleast be able to draw the table quickly. This is certainly possible with a little bit of practice.
Since the details of the basic framework for solving this type of question is covered in the article GMAT Comparisons Framework, in this article, we will skip the basics and solve this question using the framework only.
The comparisons framework
Let’s start with the question statement and see in which of these ranges would the statement be true:
Is 1/x4 > 1/x2 ?
As you can see from the ranges, for the question statement to be true, x must lie between -1 and 1. We could have arrived at the same conclusion after algebra, but that would have been time consuming and also prone to mistakes.
So the question statement truly becomes:
Is 1/x4 > 1/x2 ? is same as Is -1 < x < 1 ?
Now look at statement (a)
a) 1/x > 1/x2
Lets look at the ranges where this is true:
As you can see in the diagram, this is only possible when x>1. So given that 1/x > 1/x2, we are given that x>1. Hence x cannot be between -1 and 1. So this answers a clear “NO” to the asked question in the question statement.
Hence, statement (a) is SUFFICIENT.
Lets look at statement (b)
b) x2 > 7
Using a little bit of algebra, we get
x2 – 7 > 0 => (x-⎷7)(x+⎷7) > 0
This gives us:
either x>⎷7 OR x<-⎷7 . This clearly means that x is NOT in the range -1<x<1. Hence, this gives a clear “NO” to the asked question.
Hence, statement (a) is SUFFICIENT.
Solve GMAT Inequalities Problem Using Algebra
[Coming Soon][su_quote]Coming soon![/su_quote]